Triple Integrals in cylindrical and spherical coordinates [12.8]
Cylindrical coordinates
The figure shown is very approximately a "box" with volume
$$dV=(r\,d\theta)(dr)(dz)=r\,dr\,d\theta\,dz.$$
Spherical Polar coordinates
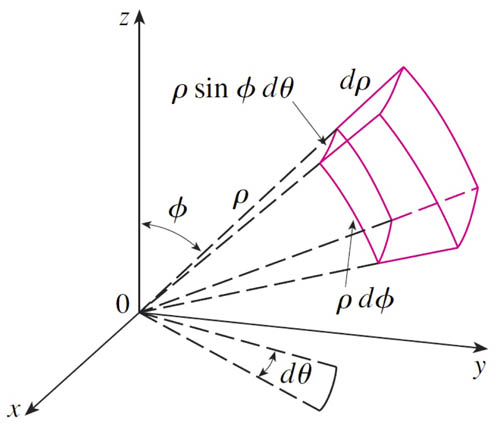The figure shown is very approximately a "box" with volume
$$dV=(\rho\,d\phi)(\rho\sin\phi\,d\theta)(d\rho)=\rho^2\sin\phi\,d\rho\,d\theta\,d\phi.$$
Examples
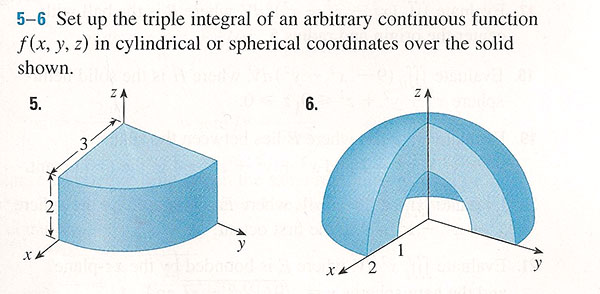
Todo
Problem 41. Total work (energy) needed to create Mt Fuji ~Right circular cone with...
- Radius of bottom=62,000 ft ~ 19 km=19,000m.
- Height of 12,400ft=3780m.
- Density of 200 lbs/ft^3 = 3,200 kg/m^3.
- Force of gravity (weight)=$mg$, where $m$ is mass (kg) and $g\approx 10$ m/s^2. If $h$ (height) has units of meters, then $mgh$ will have units of Joules.
It may be easiest to set up the integral as if you were only interested in the volume of Mt Fuji first, and then add the energy after that.
Sketch:
A large coal power plant, or nuclear plant, has power output of 2 gigawatts=2,000 megawatts=$2\times10^{9}$Joules/second: In a year of continuous operation it puts out $6.3\times 10^{16}$J.
How many months / years would such a power plant need to operate to produce the energy content you calculated above?