[13.2] - Scalar Line Integrals

1. Let $f(x,y,z)=x-3y^2+z$.
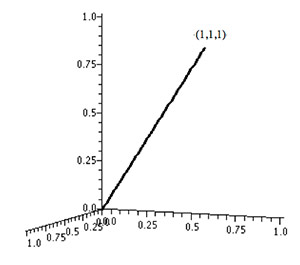
   Integrate $f$ over the line segment $C$ joining the origin $(0,0,0)$ and the point $(1,1,1)$.
   
   
   
2. Let $f(x,y,z)=x-3y^2+z$.
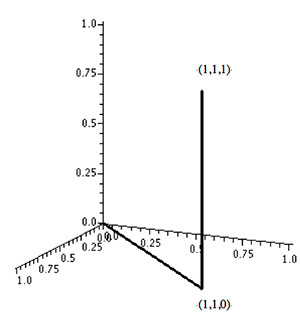
   Integrate $f$ over the 2-segment path shown joining the origin $(0,0,0)$ and the point $(1,1,1)$.
   
   

Answers

1. The line segment $C$ can be specified by $\myv r(t)=t\uv i+t\uv j+t\uv k$.

   $\myv r'(t)=\langle 1,1,1\rangle \Rightarrow |\myv r'(t)|=\sqrt 3$. And so, $$\begineq \int_C f(x,y,z)\,dx&=& \int_0^1 (t-3t^2+t)\,\sqrt 3\,dt\\ &=&\sqrt 3\int_0^1(2t-3t^2)=\sqrt 3(1^2-1^3)=0\endeq$$
   
   
   

2. The path consists of 2 segment, I'll call these $C_1: \ (0,0,0)\to(1,1,0)$ and $C_2:\ (1,1,0)\to(1,1,1)$. Then $$\int_C f\,ds=\int_{C_1}f\,ds+\int_{C_2}f\,ds.$$

   $C_1$:

   $\myv r(t)=\langle t,t,0 \rangle$ with $0\leq t\leq 1$
   $\myv r'(t)=\langle 1,1,0\rangle$ and $|\myv r'(t)|=\sqrt 2$.
   $f(x(t),y(t),z(t))= t-3t^2$
   $$\int_{C_1}f\,ds=\int_0^1 (t-3t^2)\,\sqrt 2\,dt=\left(\frac{1^2}2-(1)^3\right)\sqrt 2=-\frac{\sqrt2}2.$$

   $C_2$:

   $\myv r(t)=\langle 1,1,z \rangle$ with $0\leq z\leq 1$
   $\myv r'(t)=\langle 0,0,1\rangle$ and $|\myv r'(t)|=1$.
   $f(x(t),y(t),z(t))= 1-3(1)^2+t=t-2$
   $$\int_{C_2}f\,ds=\int_0^1 (-2+z)\,dz=\left(-2*(1)+\frac12(1)\right)=-\frac32.$$
   
   
   

   $C=C_1+C_2$:

   $$\int_C f\,dS=\frac{-\sqrt 2}{2}-\frac32=\frac{-3-\sqrt 2}2.$$